Solved Problems

Tag: source_division

  • Solve Using Current Division Rule

    Solve Using Current Division Rule

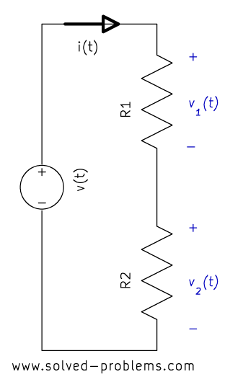
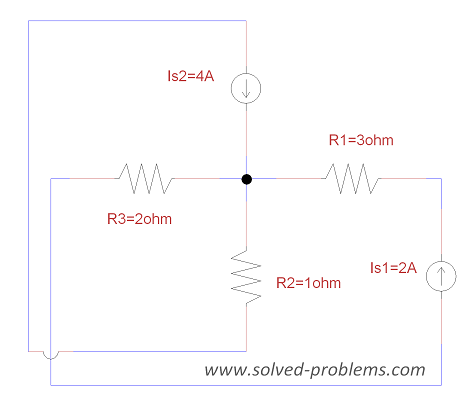
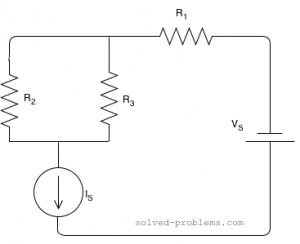
    Find current of resistors, use the current division rule.
    Problem 1246 (1)
    Suppose that R_1=2 \Omega, R_2=4 \Omega, R_3=1 \Omega, I_S=5 A and V_S=4 V

    Solution:
    R_2 and R_3 are parallel. The current of I_S is passing through them and it is actually divided between them. The branch with lower resistance has higher current because electrons can pass through that easier than the other branch. Using the current division rule, we get
    (more…)

  • Voltage Divider – Voltage Division Rule

    Voltage Divider – Voltage Division Rule

    The voltage division rule (voltage divider) is a simple rule which can be used in solving circuits to simplify the solution. Applying the voltage division rule can also solve simple circuits thoroughly. The statement of the rule is simple:

    Voltage Division Rule: The voltage is divided between two series resistors in direct proportion to their resistance.

    It is easy to prove this. In the following circuit

    Voltage Divider
    Voltage Divider

    the Ohm’s law implies that
    v_1(t)=R_1 i(t) (I)
    v_2(t)=R_2 i(t) (II)
    (more…)

  • Problem 1-16: Voltage Divider

    Problem 1-16: Voltage Divider

    Find V_x (or v_x(t)) and I_x (or i_x) using voltage division rule.
    a)
    Voltage Divider Problem - A
    b)
    Voltage Divider Problem - B
    c)
    Voltage Divider Problem - C
    d)
    Voltage Divider Problem - D

    Solution

    a)
    Voltage Divider Problem - A
    Voltage divider: V_x=\frac{5\Omega}{2\Omega+5\Omega}\times 14 V=10 V
    Ohm’s law: I_x=\frac{V_x}{5 \Omega}=2 A
    (more…)

  • Problem 1-7: Circuit Reduction – Current Divider

    Problem 1-7: Circuit Reduction – Current Divider

    Find I_9 (Hint: use circuit reduction).
    All resistors are 10\Omega and Is_1=10A
    Problem 1-7 - Circuit Reduction

    Solution
    Let’s redraw the circuit:
    (more…)