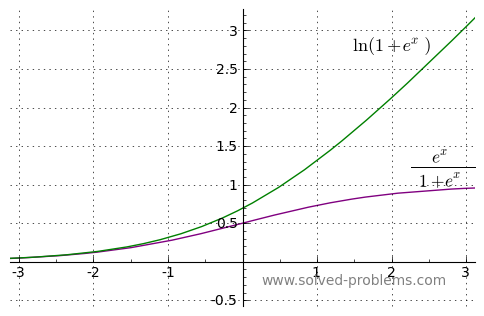
Problem 2-8: Integration

Posted byYaz 3 Comments on Problem 2-8: Integration


Find \displaystyle\int \! \frac{e^x}{1+e^x}\, dx.

Solution

Let u=1+e^x, therefore du=e^x dx \to dx=\frac{1}{u-1}du. Hence

\displaystyle\int \! \frac{e^x}{1+e^x}\, dx = \displaystyle\int \! \frac{u-1}{u} \frac{1}{u-1}\,du = \displaystyle\int \! \frac{1}{u} \,du=\ln (u)=\ln(1+e^x)
Problem 2-8

Published by Yaz

Hi! Yaz is here. I am passionate about learning and teaching. I try to explain every detail simultaneously with examples to ensure that students will remember them later too.

Join the Conversation

3 Comments

  1. Reply

  2. i really appreciate if u can send me newsletter every week. i am a pre college student , and i am glad to have this kind of website that would enable me and other fellas to succed on their academic activities.

    Reply
    1. Reply

Leave a comment

Your email address will not be published. Required fields are marked *

This site uses Akismet to reduce spam. Learn how your comment data is processed.