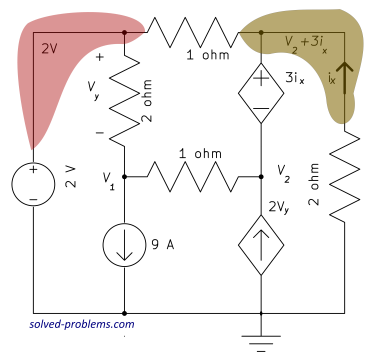
Solve the circuit with the nodal analysis and determine ![]() and
and ![]() .
.

Solution
1) Identify all nodes in the circuit. Call the number of nodes ![]() .
.
The circuit has 5 nodes. Therefore, ![]() .
.
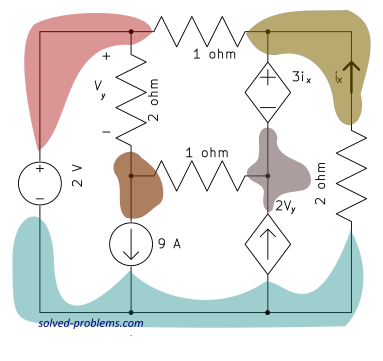
2) Select a reference node. Label it with reference (ground) symbol.
The node at the bottom is the best candidate. It is the node with largest number of elements connected to it.
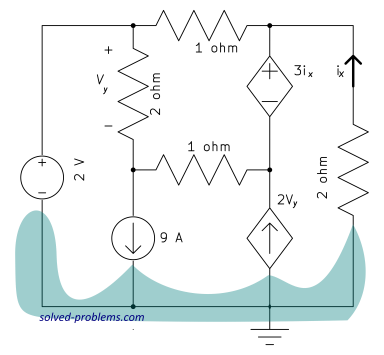
3) Assign a variable for each node whose voltage is unknown.
Four nodes are remaining:
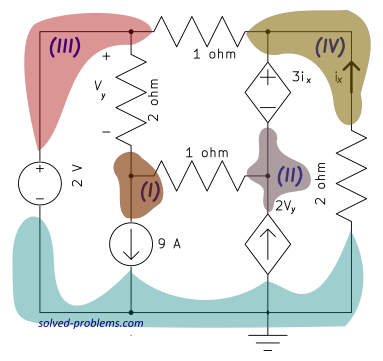
Node I is a regular node. A dependent voltage source is located between node II and node III. Therefore, node II and node III form a supernode. Node IV is connected to a voltage source whose other node is the reference node. We label the voltages of node I and node II with ![]() and
and ![]() respectively.
respectively.
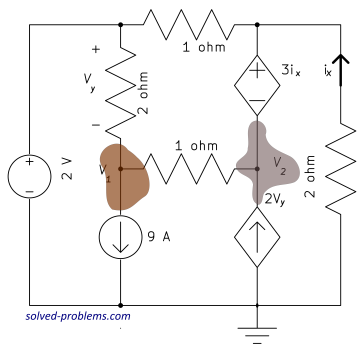
For node IV, the ![]() voltage source provides the voltage of the node. Since the positive terminal of the voltage source is connected to the node, the node voltage is
voltage source provides the voltage of the node. Since the positive terminal of the voltage source is connected to the node, the node voltage is ![]() .
.
4) If there are dependent sources in the circuit, write down equations that express their values in terms of other node voltages.
The voltage of node III is ![]() . On the other hand
. On the other hand ![]() is the current of the
is the current of the ![]() resistor in the right hand side. Using the Ohm’s law:
resistor in the right hand side. Using the Ohm’s law:
voltage of the ![]() resistor =
resistor = ![]() . (Eq. 1)
. (Eq. 1)
Please note that the direction of ![]() is from the reference node to node III. Since it is always assumed that the reference node is the negative terminal for the defined node voltages, the voltage of node III is
is from the reference node to node III. Since it is always assumed that the reference node is the negative terminal for the defined node voltages, the voltage of node III is ![]() (instead of
(instead of ![]() ).
).
By solving Eq. 1:
![]() .
.
Consequently, the voltage of node III is ![]() .
.
Note that we always write unknowns in terms of node voltages in nodal analysis.

Note that ![]() .
. ![]() is the current of the dependent current source. Now, we need to find the current of the dependent current source in terms of the node voltages.
is the current of the dependent current source. Now, we need to find the current of the dependent current source in terms of the node voltages. ![]() is the voltage across node IV and Node I. The positive terminal is connected to node IV and the negative one is connected to node II. Therefore,
is the voltage across node IV and Node I. The positive terminal is connected to node IV and the negative one is connected to node II. Therefore, ![]() . You can verify this by applying KVL around the loop consisting of the reference node, node I and node IV.
. You can verify this by applying KVL around the loop consisting of the reference node, node I and node IV.
5) Write down a KCL equation for each node by setting the total current flowing out of the node to zero.
For node I:
![]() .
.
![]() (Eq. 2)
(Eq. 2)
For supernode II&III:
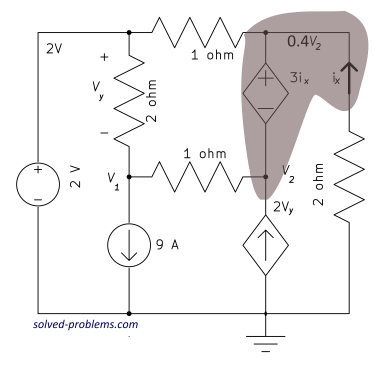
![]() . Substituting
. Substituting ![]() and simplifying:
and simplifying:
![]() . (Eq. 3)
. (Eq. 3)
Solving the set of equations Eq. 2 and Eq. 3 results in ![]() and
and ![]() . Therefore
. Therefore ![]() and
and ![]() .
.
Leave a Reply